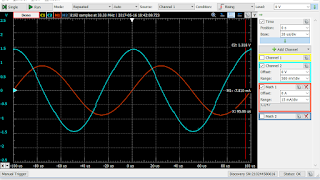
5/25/2017 Classroom Activities+ "Apparent Power and Power Factor " Lab

5/25/2017

We derived how to find effective current, which at the end is equal to rms current, and finding amplitude of voltage given the value of Vrms

Practice on parametrizing a curve:

Finding Irms, apparent power, and power factor of a circuit

Finding complex power:

"Apparent Power and Power Factor " Lab

Prelab:

Waveform on the circuit with the second resistor having the value of 10 ohm

Waveform on the circuit with the second resistor having the value of 47 ohm

Waveform on the circuit with the second resistor having the value of 10 ohm

 Comparing the theoretical and experimental values

On the circuit with the second resistor having the value of 10 ohm

On the circuit with the second resistor having the value of 47 ohm

On the circuit with the second resistor having the value of 100 ohm

Summary:

We could calculate I rms and V rms by divinding I and V with square root of 2 respectively. We could calculate the average power by multiplying Irms and Vrms. We could also calculate the power factor by calculating the ratio of the real part of the power to the magnitude of the complex power., and taking the arc cosine of the angle. Also, there is a "measure" option in waveform that calculates the rms value while collecting data.

5/23/2017 + "Op-Amp relaxation Oscillator" Lab

5/23/2017

Problem involving op amp and using nodal analysis to solve it

We were then assigned to find the output voltage of an op-amp with no open loop gain

Finding instaneous power

Finding current and the equivalent impedance of a circuit

"Op-Amp relaxation Oscillator" Lab

Prelab(finding a combination of resistor and capacitorthat would output a frequency of one's last 3 digit on student id):

Everycircuit on the proposed resistor and capacitor combo:

The circuit:

The components:

Waveform of the voltage across the 7.3k resistor

Waveform of the voltage across the 7.3k resistor(yellow) and the capacitor(blue)

Waveform of the voltage across the two 1k resistor(yellow) and the capacitor(blue)

Values comparing the theoretical and experimental frequency

Summary:

Everycircuit has the "inject noise" option, where by dragging and shaking the circuit, it allows us to make a circuit without a power supply and still read its values (circuits with capacitors and op amp inside). From the experiment, we could see that the theoretical and measured frequency across the 7.3k resistor(the one we fiddled with) are pretty close to each other. It shows that we could create a circuit with a desired frequency by changing the combination of the capacitor and the feedback resistor. Also, by leaving the two resistance near the ground to be equal to each other, we make the calculation for the desired frequency much easier for ourselves.

5/18/2017 Classroom Activities

5/18/2017

To start the day, we were told to list the different methods of circuit analysis that we learned so far

We then went to practice using KCL on AC circuit

After that, we practiced using KVL on AC circuit

Next up is using superposition as the method of circuit analysis

Finding the Thevenin voltage of a circuit

Finding Thevenin Resistance of a circuit

Summary: 
All the previously learned circuit analysis techniques that we learn for DC also works for AC.
**No lab today**

5/16/2017 Classroom Activities + "Impedance" Lab

5/16/2017

We started the day by drawing a phasor diagram and how we think it would look like for resistor, capacitor, and inductor

We then find the impedance of elements when the angular frequency approaches infinity

We also practiced on finding current of a circuit with a voltage source

After the lab, we went on to find the equivalent impedance of a circuit

We also did a problem finding an equivalent impedance and also finding the voltage across one of the elements

 

"Impedance" Lab

Prelab:

Waveform for the Resistor as the second element:

at f=1kHz

at f=5kHz

at f=10kHz

Waveform for the Capacitor as the second element:

at f=1kHz

at f=5kHz

at f=10kHz

Waveform for the Inductor as the second element:

at f=1kHz

at f=5kHz

at f=10kHz

Values:

Summary:

As predicted, current and voltage are in phase for resistors, current leads voltage for capacitors, and current lags voltage for inductors.  For Inductors, the phase difference increases as angular frequency increases, and decreases as angular frequency decreases. It is the opposite for capacitors; phase difference decreases as angular frequency increases, and increases as angular frequency decreases. The theoretical and experimental values are pretty close to each other, which means that the formula used to find impedance of the elements holds true.

5/11/2017 Classroom Activities + "Phasors: Passive RL Circuit Response " Lab

5/11/2017

Today we went over how to rewrite sinusoids into phasors and exponential.

Practice problem on adding sinusoids

Practice problem on converting exponential to phasor using eulers method

Practice problem on converting rectangular to polar

Practice problem on converting sinusoid to phasor

Practice problem on finding current in different forms

Practice problem on Graphical Representation method of addition

Practice problem on finding out which equation is leading

Practice problem on solving problem that requires some conversion back and forth

"Phasors: Passive RL Circuit Response " Lab

Prelab:

measuring the resistance

Waveform Graph:


at omega=10 omega_c (Blue is V_n, red is I_n)

at omega=omega_c /10 (Blue is V_n, red is I_n)

at omega= omega_c (Blue is V_n, red is I_n)

Comparing theoretical and experimental values:

Summary:

We could see how the voltage leads the current in an RL circuit from the waveform graphs above. We also could see that by adjusting the angular frequency of the signal, we are also changing the phase difference between the current and the voltage in RL circuit.  From the experiment conducted, when we increase the angular frequency, the phase difference between the voltage and the current increases, while when we decrease the angular frequency, the phase difference also goes down. The gain has a different trend, where it decreases as angular frequency is increased, and increases as angular frequency is decreases. Both of the trend are in agreement with the formulas that we used/derived.